The Regression Below Predicts the Daily Number of Skiers Who $F$

question 18

Essay

The regression below predicts the daily number of skiers who visit a small ski resort based on three explanatory variables.
The data is a random sample of 30 days from the past two ski seasons. The variables are: SKIERS the number of skiers who visit the resort on that day
SNOW the number of inches of snow on the ground
TEMP the high temperature for the day in degrees $F$ .
WEEKDAY an indicator variable, weekday $= 1$ , weekend $= 0$
Dependent variable is Skiers
R squared $= 25.4 \% \quad$ R squared (adjusted) $= 16.8 \%$
$\mathrm { s } = 125.1$ with $30 - 4 = 26$ degrees of freedom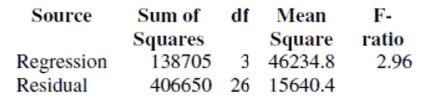


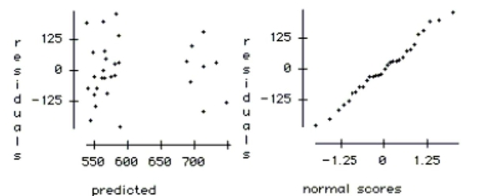
$\begin{array} { l r r r r } \text { Variable } & \text { Coefficient } & \text { SE(Coeff) } & \text { t-ratio } & \text { p-value } \\ \text { Constant } & 559.869 & 76.78 & 7.29 & < 0.0001 \\ \text { Snow } & 1.424 & 2.70 & 0.53 & 0.6019 \\ \text { Temp } & - 1.604 & 2.77 & - 0.58 & 0.5677 \\ \text { Weekend } & 147.349 & 51.86 & 2.84 & 0.0086 \end{array}$


-Which of the explanatory variables appear to be associated with the number of skiers, and
which do not? Explain how you reached your conclusion.

---

Definitions:

Compliment Close

A close wherein the salesperson ends with a compliment to the prospect.

Alternative-Choice

Alternative-choice is a sales technique that presents the buyer with a choice between two or more options, aiming to facilitate a decision.

Freezer

An appliance or industrial facility used for the cold storage of goods, especially foodstuffs, to preserve them over time.

Summarize Benefits

Involves condensing key advantages or positive aspects of a product, service, or situation into a brief, clear presentation.