Here are data about the average January low temperature in cities in the United States, and factors that might allow us to
predict temperature. The data, available for 55 cities, include:  We will attempt to make a regression model to help account for mean January temperature and to understand the effects of
We will attempt to make a regression model to help account for mean January temperature and to understand the effects of
the various predictors.
At each step of the analysis you may assume that things learned earlier in the process are known.
Units Note: The "degrees" of temperature, given here on the Fahrenheit scale, have only coincidental language relationship to
the "degrees" of longitude and latitude. The geographic "degrees" are based on modeling the Earth as a sphere and dividing it
up into 360 degrees for a full circle. Thus 180 degrees of longitude is halfway around the world from Greenwich, England
(0°) and Latitude increases from 0 degrees at the Equator to 90 degrees of (North) latitude at the North Pole. 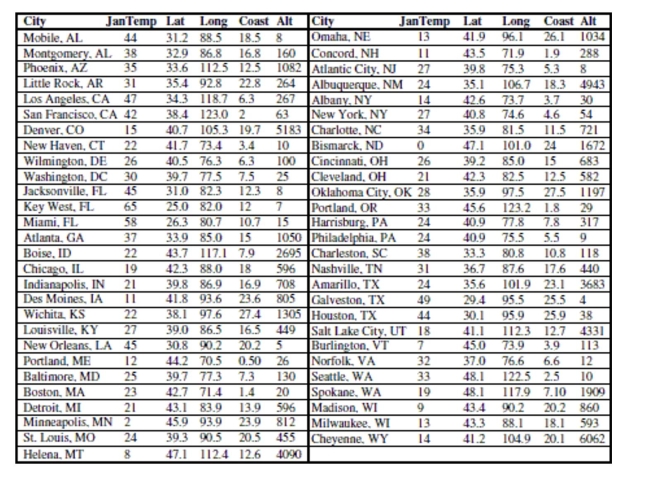
-Here is the regression with both Latitude and Longitude as predictors: Dependent variable is: JanTemp R squared R squared (adjusted)
with degrees of freedom
The coefficient of Long in this regression differs from the coefficient of Long in the simple regression of JanTemp on Long. What is the meaning of the coefficient of Long in this regression? Are you confident (at ) that the coefficient is not zero? Why or why not?
Definitions:
Taxable Wage Base
The maximum amount of an employee's earnings that are subject to certain taxes, such as Social Security tax, within a given tax year.
Employer's Portion
The part of employment taxes and benefits that the employer is responsible for paying, separate from employee withholdings.
Taxes
Mandatory financial contributions imposed by a government on individuals, corporations, and other entities to fund public expenditures.
FICA
FICA stands for the Federal Insurance Contributions Act and refers to the taxes collected to fund Social Security and Medicare programs in the United States.
Q24: An observed frequency distribution is as
Q33: Find and interpret a 95% confidence interval
Q39: A survey of some AP* Stats students
Q54: Gun control Two friends who strongly disagree
Q59: A survey conducted in a small
Q71: State your conclusion.
Q74: An electronics store receives a shipment of
Q77: <span class="ql-formula" data-value="\begin{array}{r|r|r|r|r|r|r|r|r}\mathrm{x} & 0.55 & 0.33
Q99: Internet access A recent Gallup poll found
Q154: Bill kept track of the number