Solved

TABLE 16-12 A Local Store Developed a Multiplicative Time-Series Model to Forecast

question 40

Multiple Choice

TABLE 16-12
A local store developed a multiplicative time-series model to forecast its revenues in future quarters, using quarterly data on its revenues during the 4-year period from 2005 to 2009. The following is the resulting regression equation:
log₁₀ 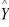= 6.102 + 0.012 X - 0.129 Q₁ - 0.054 Q₂ + 0.098 Q₃
where 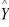is the estimated number of contracts in a quarter.
X is the coded quarterly value with X = 0 in the first quarter of 2005.
Q₁ is a dummy variable equal to 1 in the first quarter of a year and 0 otherwise.
Q₂ is a dummy variable equal to 1 in the second quarter of a year and 0 otherwise.
Q₃ is a dummy variable equal to 1 in the third quarter of a year and 0 otherwise.
-Referring to Table 16-12, in testing the significance of the coefficient for Q₁ in the regression equation (-0.129) which has a p-value of 0.492. Which of the following is the best interpretation of this result?

Definitions:

Scatterplot

A graphical representation using dots to show the relationship between two quantitative variables.

Correlation

A gauge that determines the extent of simultaneous variations between two or more variables.

Lurking Variable

A variable that is not directly measured but affects the variables of interest in a study, potentially confounding the results.

Heights

Refers to the measurement of vertical length, either of an individual or object, typically measured from base to top.