An Actuary Wanted to Develop a Model to Predict How

question 178

Essay

An actuary wanted to develop a model to predict how long individuals will live. After consulting a number of physicians, she collected the age at death (y), the average number of hours of exercise per week (x1), the cholesterol level (x2), and the number of points that the individual's blood pressure exceeded the recommended value (x3). A random sample of 40 individuals was selected. The computer output of the multiple regression model is shown below. 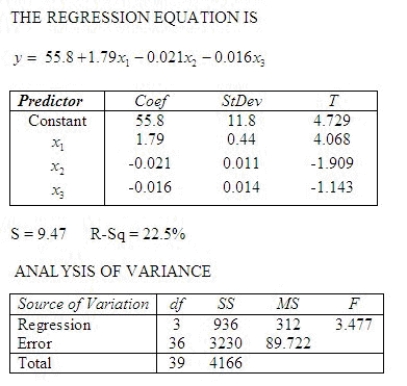Is there enough evidence at the 10% significance level to infer that the model is useful in predicting length of life?
______________ enough evidence at the 10% significance level to infer that the model is useful in predicting length of life.
Is there enough evidence at the 1% significance level to infer that the average number of hours of exercise per week and the age at death are linearly related?
What is the test statistic?
t = ______________
Conclude:
______________ .
There ______________ enough evidence at the 1% significance level to infer that the average number of hours of exercise per week and the age at death are linearly related.
Is there enough evidence at the 5% significance level to infer that the cholesterol level and the age at death are negatively linearly related?
What is the test statistic?
t = ______________
Conclude:
______________ .
There ______________ enough evidence at the 5% significance level to infer that the cholesterol level and the age at death are negatively linearly related.
Is there sufficient evidence at the 5% significance level to infer that the number of points that the individual's blood pressure exceeded the recommended value and the age at death are negatively linearly related?
What is the test statistic?
t = ______________
Conclude:
______________ .
There ______________ sufficient evidence at the 5% significance level to infer that the number of points that the individual's blood pressure exceeded the recommended value and the age at death are negatively linearly related.
What is the coefficient of determination? ______________
Explain:
________________________________________________________

---

Definitions:

Neurocognitive Disorder

A class of mental health disorders that primarily affect cognitive abilities including memory, problem-solving, and attention.

Age-related

Pertaining to changes or phenomena that occur in an organism as it ages, including physical, cognitive, and emotional aspects.

Neuroimaging Studies

Research involving the use of various techniques to directly or indirectly image the structure, function, or pharmacology of the nervous system.

Brain Deterioration

A process where cognitive functions and neuronal health decline, often due to aging or neurological diseases.