SCENARIO 18-10 Given Below Are Results from the Regression Analysis

question 153

True/False

SCENARIO 18-10 Given below are results from the regression analysis where the dependent variable is the number of weeks a worker is unemployed due to a layoff (Unemploy)and the independent variables are the age of the worker (Age), the number of years of education received (Edu), the number of years at the previous job (Job Yr), a dummy variable for marital status (Married: 1 = married, 0 = otherwise), a dummy variable for head of household (Head: 1 = yes, 0 = no)and a dummy variable for management position (Manager: 1 = yes, 0 = no).We shall call this Model 1.The coefficient of partial determination of each o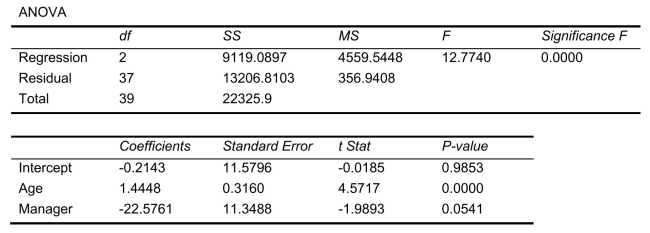f the 6 predictors are, respectively, 0.2807, 0.0386, 0.0317, 0.0141, 0.0958, and 0.1201. 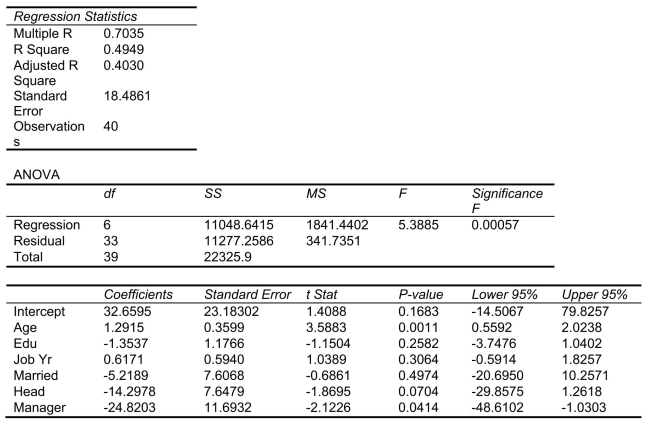Model 2 is the regression analysis where the dependent variable is Unemploy and the independent variables are Age and Manager.The results of the regression analysis are given below: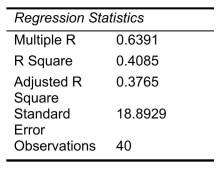
-Referring to Scenario 18-10 Model 1, we can conclude that, holding constant the effect of the other independent variables, the number of years of education received has no impact on the mean number of weeks a worker is unemployed due to a layoff at a 1% level of significance if all we have is the information of the 95% confidence interval estimate for .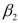

---

Definitions:

Bulimia

An eating disorder marked by binge eating followed by methods to avoid weight gain, such as self-induced vomiting, fasting, or excessive exercise.

Young People's Health

Refers to the physical, mental, and social well-being of individuals in their late childhood, adolescence, and early adulthood.

Marijuana

A psychoactive drug derived from the Cannabis plant, used for recreational or medicinal purposes.

Adolescents

Individuals in the developmental stage bridging childhood and adulthood, typically ranging from the early teenage years to the early twenties.