Use This Information,along with Its Associated Sensitivity Report,to Answer the Following

question 44

Multiple Choice

Use this information,along with its associated Sensitivity Report,to answer the following questions.
A production manager wants to determine how many units of each product to produce weekly to maximize weekly profits.Production requirements for the products are shown in the following table.
$$\begin{array} { | c | c | c | c | } \hline \underline { \text { Product } } & \frac { \text { Material 1 } } { ( \mathrm { lbs } ) } & \frac { \text { Material 2 } } { ( \mathrm { lbs } . ) } & \text { Labor (hours) } \\\hline \underline { \underline { \mathrm { A } } } & \underline { 3 } & \underline { 2 } & \underline { 4 } \\\hline \underline { \mathrm { B } } & \underline { 1 } & \underline { 4 } & \underline { 2 } \\\hline \underline { \mathrm { C } } & \underline { 5 } & \underline { \text { none } } & \underline { 3.5 } \\\hline\end{array}$$
Material 1 costs $7 a pound,material 2 costs $5 a pound,and labor costs $15 per hour.Product A sells for $101 a unit,product B sells for $67 a unit,and product C sells for $97.50 a unit.Each week there are 300 pounds of material 1;400 pounds of material 2;and 200 hours of labor.The output of product A should not be more than one-half of the total number of units produced.Moreover,there is a standing order of 10 units of product C each week.
$$\begin{array}{l}\text { Formulation }\\\begin{array} { l l } \ { \text { Max } } & 10 \mathrm {~A} + 10 \mathrm {~B} + 10 \mathrm { C } \\\text { Subject to: } & \\& 3 \mathrm {~A} + \mathrm { B } + 5 \mathrm { C } \leq 300 \text { (constraint \#1) } \\& 2 \mathrm {~A} + 4 \mathrm {~B} \leq 400 \text { (constraint \#2) } \\& 4 \mathrm {~A} + 2 \mathrm {~B} + 3.5 \mathrm { C } \leq 200 \text { (constraint \#3) } \\& \mathrm { C } \geq 10 \text { (constraint \#4) } \\& \mathrm { A } , \mathrm { B } , \mathrm { C } \geq 0\end{array}\end{array}$$

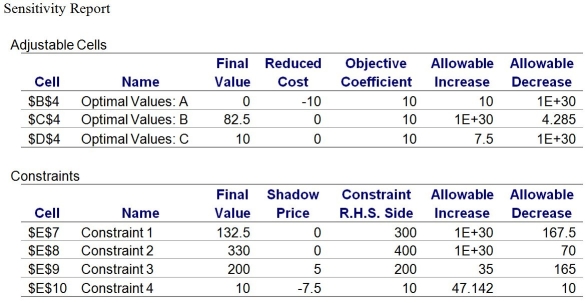
-Suppose that we force the production of one unit of product A.The new objective function value will be

---

Definitions:

Employment Act of 1946

A United States federal law aiming to promote maximum employment, production, and purchasing power through economic stability policies.

Aggregate Demand

The total demand for final goods and services in an economy at a given time.

Theory of Liquidity Preference

A theory suggesting that individuals prefer to have their resources in liquid forms and how that preference influences interest rates.

Interest Rates

The cost of borrowing money, expressed as a percentage of the amount borrowed, paid by the borrower to the lender for using their money.