Examlex

Solved

The Following Table Shows the Annual Revenues (In Millions of Dollars)

question 16

Essay

The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The autoregressive models of order 1 and 2, y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + ε<sub>t</sub>, and y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + β<sub>2</sub>y<sub>t - 2</sub> + ε<sub>t</sub>, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. Model AR(1): Model AR(2): When for AR(1), H<sub>0</sub>: β<sub>0</sub> = 0 is tested against H<sub>A</sub>: β<sub>0</sub> ≠ 0, the p-value of this t test shown the output as 0.9590. This could suggest that the model y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1 </sub>+ ε<sub>t</sub> might be an alternative to the AR(1) model y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t-1</sub> + ε<sub>t</sub>. Partial output for this simplified model is as follows: Find the revenue forecast for 2012 through the use of y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>. The autoregressive models of order 1 and 2, yt = β0 + β1yt - 1 + εt, and yt = β0 + β1yt - 1 + β2yt - 2 + εt, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below.
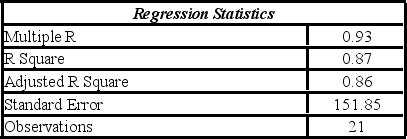
Model AR(1): The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The autoregressive models of order 1 and 2, y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + ε<sub>t</sub>, and y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + β<sub>2</sub>y<sub>t - 2</sub> + ε<sub>t</sub>, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. Model AR(1): Model AR(2): When for AR(1), H<sub>0</sub>: β<sub>0</sub> = 0 is tested against H<sub>A</sub>: β<sub>0</sub> ≠ 0, the p-value of this t test shown the output as 0.9590. This could suggest that the model y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1 </sub>+ ε<sub>t</sub> might be an alternative to the AR(1) model y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t-1</sub> + ε<sub>t</sub>. Partial output for this simplified model is as follows: Find the revenue forecast for 2012 through the use of y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>. 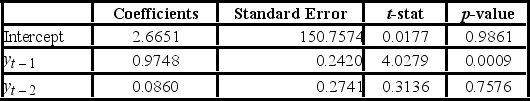The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The autoregressive models of order 1 and 2, y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + ε<sub>t</sub>, and y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + β<sub>2</sub>y<sub>t - 2</sub> + ε<sub>t</sub>, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. Model AR(1): Model AR(2): When for AR(1), H<sub>0</sub>: β<sub>0</sub> = 0 is tested against H<sub>A</sub>: β<sub>0</sub> ≠ 0, the p-value of this t test shown the output as 0.9590. This could suggest that the model y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1 </sub>+ ε<sub>t</sub> might be an alternative to the AR(1) model y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t-1</sub> + ε<sub>t</sub>. Partial output for this simplified model is as follows: Find the revenue forecast for 2012 through the use of y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>. 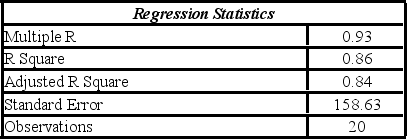Model AR(2): The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The autoregressive models of order 1 and 2, y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + ε<sub>t</sub>, and y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + β<sub>2</sub>y<sub>t - 2</sub> + ε<sub>t</sub>, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. Model AR(1): Model AR(2): When for AR(1), H<sub>0</sub>: β<sub>0</sub> = 0 is tested against H<sub>A</sub>: β<sub>0</sub> ≠ 0, the p-value of this t test shown the output as 0.9590. This could suggest that the model y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1 </sub>+ ε<sub>t</sub> might be an alternative to the AR(1) model y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t-1</sub> + ε<sub>t</sub>. Partial output for this simplified model is as follows: Find the revenue forecast for 2012 through the use of y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>. 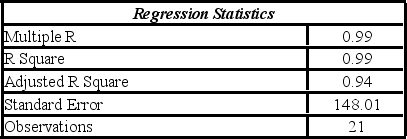The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The autoregressive models of order 1 and 2, y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + ε<sub>t</sub>, and y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + β<sub>2</sub>y<sub>t - 2</sub> + ε<sub>t</sub>, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. Model AR(1): Model AR(2): When for AR(1), H<sub>0</sub>: β<sub>0</sub> = 0 is tested against H<sub>A</sub>: β<sub>0</sub> ≠ 0, the p-value of this t test shown the output as 0.9590. This could suggest that the model y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1 </sub>+ ε<sub>t</sub> might be an alternative to the AR(1) model y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t-1</sub> + ε<sub>t</sub>. Partial output for this simplified model is as follows: Find the revenue forecast for 2012 through the use of y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>. When for AR(1), H0: β0 = 0 is tested against HA: β0 ≠ 0, the p-value of this t test shown the output as 0.9590. This could suggest that the model yt = β1yt-1 + εt might be an alternative to the AR(1) model yt = β0 + β1yt-1 + εt. Partial output for this simplified model is as follows: The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The autoregressive models of order 1 and 2, y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + ε<sub>t</sub>, and y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + β<sub>2</sub>y<sub>t - 2</sub> + ε<sub>t</sub>, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. Model AR(1): Model AR(2): When for AR(1), H<sub>0</sub>: β<sub>0</sub> = 0 is tested against H<sub>A</sub>: β<sub>0</sub> ≠ 0, the p-value of this t test shown the output as 0.9590. This could suggest that the model y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1 </sub>+ ε<sub>t</sub> might be an alternative to the AR(1) model y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t-1</sub> + ε<sub>t</sub>. Partial output for this simplified model is as follows: Find the revenue forecast for 2012 through the use of y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>. The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The autoregressive models of order 1 and 2, y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + ε<sub>t</sub>, and y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + β<sub>2</sub>y<sub>t - 2</sub> + ε<sub>t</sub>, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. Model AR(1): Model AR(2): When for AR(1), H<sub>0</sub>: β<sub>0</sub> = 0 is tested against H<sub>A</sub>: β<sub>0</sub> ≠ 0, the p-value of this t test shown the output as 0.9590. This could suggest that the model y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1 </sub>+ ε<sub>t</sub> might be an alternative to the AR(1) model y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t-1</sub> + ε<sub>t</sub>. Partial output for this simplified model is as follows: Find the revenue forecast for 2012 through the use of y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>. Find the revenue forecast for 2012 through the use of yt = β1yt-1 + εt.

Definitions:

Thalamus

A brain structure acting as a relay station for sensory and motor signals to the cerebral cortex, excluding the sense of smell.

Relay Information

The process of transmitting information or messages from one person, device, or group to another.

Coordinate Muscle

The process of different muscles working together to perform movements smoothly and accurately.

Regulate Hormones

The process by which the body maintains hormonal balance, involving the production, secretion, and breakdown of hormones that influence various bodily functions.

Related Questions

Q1: A researcher gathers data on 25 households

Q26: The following data show the demand for

Q41: An over-the-counter drug manufacturer wants to examine

Q64: A company that produces financial accounting software

Q64: The accompanying table shows the regression results

Q82: At expiry, a holder of a call

Q89: 'Novation' refers to the system of margin

Q99: The following table includes the information about

Q121: Describe the main features of option contracts.What

Q123: Explain how volatility influences option prices.