Instruction 12 $\text { Regression statistics }$ $\text { ANOVA }$

question 116

Multiple Choice

Instruction 12.10
A computer software developer would like to use the number of downloads (in thousands) for the trial version of his new shareware to predict the amount of revenue (in thousands of dollars) he can make on the full version of the new shareware. Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different sharewares that he has developed:
$\text { Regression statistics }$
$\begin{array}{|l|l|}\hline \text { MultipleR } & 0.8691 \\\hline \text { R Square } & 0.7554 \\\hline \begin{array}{l}\text { Adjusted R } \\\text { Square }\end{array} & 0.7467 \\\hline \text { Standard Error } & 44.4765 \\\hline \text { Observations } & 30.0000\\\hline \end{array}$

$\text { ANOVA }$
$\begin{array}{|l|l|l|l|l|l|}\hline & d f & S S & M S & F & \begin{array}{l}\text { Significance } \\F\end{array} \\\hline \text { Regression } & 1 & 171062.9193 & 171062.9193 & 86.4759 & 0.0000 \\\hline \text { Residual } & 28 & 55388.4309 & 1978.1582 & & \\\hline \text { Total } & 29 & 226451.3503 & & & \\\hline\end{array}$

$\begin{array}{|l|l|l|l|l|l|l|}\hline & \text { Coefficients } & \begin{array}{l}\text { Standard } \\\text { Error }\end{array} & \text { t Stat } & p \text {-value } & \text { Lower 95\% } & \text { Upper 95\% } \\\hline \text { Intercept } & -95.0614 & 26.9183 & -3.5315 & 0.0015 & -150.2009 & -39.9218 \\\hline \text { Download } & 3.7297 & 0.4011 & 9.2992 & 0.0000 & 2.9082 & 4.5513 \\\hline\end{array}$
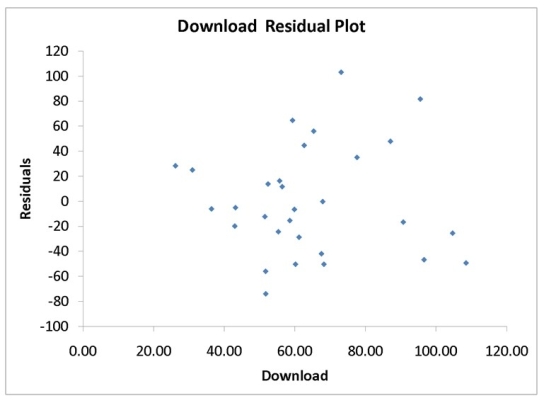
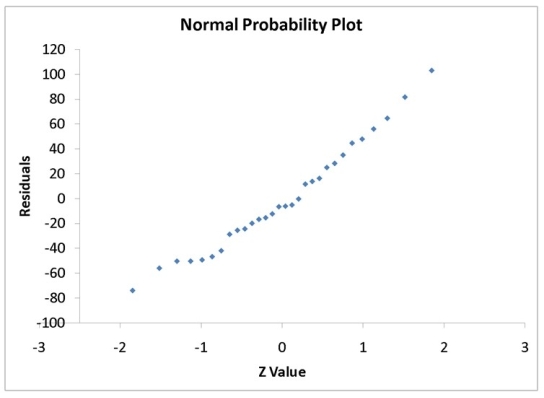
-Referring to Instruction 12.10,which of the following is the correct interpretation for the slope coefficient?

• A) For each incsrease of 1 million dollars in box office gross, expected home video units sold are estimated to increase by 4.3331 thousand units.
• B) For each increase of 1 dollar in box office gross, expected home video units sold are estimated to increase by 4.3331 units.
• C) For each increase of 1 million dollars in box office gross, expected home video units sold are estimated to increase by 4.3331 units.
• D) For each increase of 1 dollar in box office gross, expected home video units sold are estimated to increase by 4.3331 thousand units.

---

Definitions:

Foot-In-The-Door Effect

The foot-in-the-door effect is a psychological principle that suggests people are more likely to agree to a large request if they first agree to a smaller one.

Minority Influence

The process by which a smaller part of a group changes the beliefs or behaviors of the majority, often through consistency, commitment, and flexibility.

Self-Confident

Pertains to a state of being secure in oneself and one's abilities.

Groupthink

A psychological phenomenon that occurs within a group of people in which the desire for harmony or conformity results in an irrational or dysfunctional decision-making outcome.