Instruction 12 $\text { Regression statistics }$ $\text { ANOVA }$

question 58

True/False

Instruction 12.21
A computer software developer would like to use the number of downloads (in thousands) for the trial version of his new shareware to predict the amount of revenue (in thousands of dollars) he can make on the full version of the new shareware. Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different sharewares that he has developed:
$\text { Regression statistics }$
$\begin{array}{|l|l|}\hline \text { MultipleR } & 0.8691 \\\hline \text { R Square } & 0.7554 \\\hline \begin{array}{l}\text { Adjusted R } \\\text { Square }\end{array} & 0.7467 \\\hline \text { Standard Error } & 44.4765 \\\hline \text { Observations } & 30.0000\\\hline \end{array}$

$\text { ANOVA }$
$\begin{array}{|l|l|l|l|l|l|}\hline & d f & S S & M S & F & \begin{array}{l}\text { Significance } \\F\end{array} \\\hline \text { Regression } & 1 & 171062.9193 & 171062.9193 & 86.4759 & 0.0000 \\\hline \text { Residual } & 28 & 55388.4309 & 1978.1582 & & \\\hline \text { Total } & 29 & 226451.3503 & & & \\\hline\end{array}$

$\begin{array}{|l|l|l|l|l|l|l|}\hline & \text { Coefficients } & \begin{array}{l}\text { Standard } \\\text { Error }\end{array} & \text { t Stat } & p \text {-value } & \text { Lower 95\% } & \text { Upper 95\% } \\\hline \text { Intercept } & -95.0614 & 26.9183 & -3.5315 & 0.0015 & -150.2009 & -39.9218 \\\hline \text { Download } & 3.7297 & 0.4011 & 9.2992 & 0.0000 & 2.9082 & 4.5513 \\\hline\end{array}$


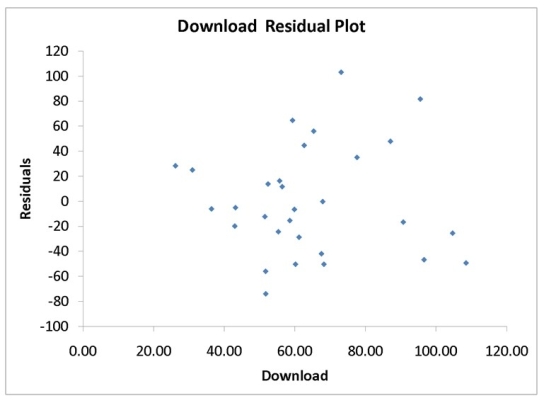
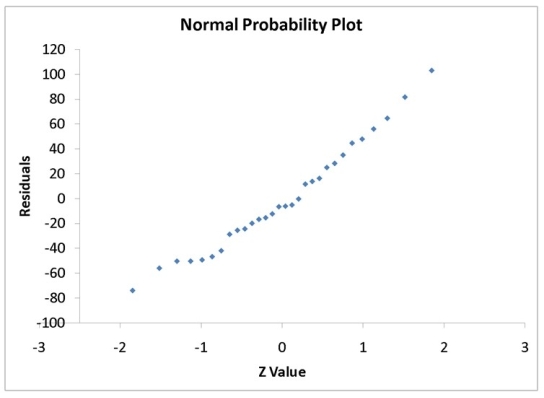
-Referring to Instruction 12.21,the normality of error assumption appears to have been violated.

Gain knowledge of policy impacts on family structure, specifically the one-child policy in China.

Grasp the development timeline for friendships and self-concept in children.

Understand sibling dynamics and their influence on early childhood development.

Understand different types of play and their significance in child development.

---

Definitions:

Arbitragers

Individuals or entities that seek to profit from price discrepancies of the same asset in different markets or forms.

Russian Rubles

The currency used in Russia, which acts as a medium of exchange within the Russian economy.

Real Exchange Rate

The real exchange rate measures the value of one currency against another, adjusted for inflation, reflecting the purchasing power of one country's currency in another country.

Arbitrage

The practice of buying and selling assets in different markets or in different forms to exploit price differences for a profit.