Earnings Functions Attempt to Find the Determinants of Earnings,using Both

question 40

Essay

Earnings functions attempt to find the determinants of earnings,using both continuous and binary variables.One of the central questions analyzed in this relationship is the returns to education.
(a)Collecting data from 253 individuals,you estimate the following relationship 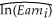= 0.54 + 0.083 × Educ,R2 = 0.20,SER = 0.445
(0.14)(0.011)
where Earn is average hourly earnings and Educ is years of education.
What is the effect of an additional year of schooling? If you had a strong belief that years of high school education were different from college education,how would you modify the equation? What if your theory suggested that there was a "diploma effect"?
(b)You read in the literature that there should also be returns to on-the-job training.To approximate on-the-job training,researchers often use the so called Mincer or potential experience variable,which is defined as Exper = Age - Educ - 6.Explain the reasoning behind this approximation.Is it likely to resemble years of employment for various sub-groups of the labor force?
(c)You incorporate the experience variable into your original regression 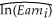= -0.01 + 0.101 × Educ + 0.033 × Exper - 0.0005 × Exper2,
(0.16)(0.012)(0.006)(0.0001)
R2 = 0.34,SER = 0.405
What is the effect of an additional year of experience for a person who is 40 years old and had 12 years of education? What about for a person who is 60 years old with the same education background?
(d)Test for the significance of each of the coefficients of the added variables.Why has the coefficient on education changed so little? Sketch the age-(log)earnings profile for workers with 8 years of education and 16 years of education.
(e)You want to find the effect of introducing two variables,gender and marital status.Accordingly you specify a binary variable that takes on the value of one for females and is zero otherwise (Female),and another binary variable that is one if the worker is married but is zero otherwise (Married).Adding these variables to the regressors results in: 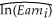= 0.21 + 0.093 × Educ + 0.032 × Exper - 0.0005 × Exper2
(0.16)(0.012)(0.006)(0.0001)
- 0.289 × Female + 0.062 Married,
(0.049)(0.056)
R2 = 0.43,SER = 0.378
Are the coefficients of the two added binary variables individually statistically significant? Are they economically important? In percentage terms,how much less do females earn per hour,controlling for education and experience? How much more do married people make? What is the percentage difference in earnings between a single male and a married female? What is the marriage differential between males and females?
(f)In your final specification,you allow for the binary variables to interact.The results are as follows: 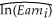= 0.14 + 0.093 × Educ + 0.032 × Exper - 0.0005 × Exper2
(0.16)(0.011)(0.006)(0.001)
- 0.158 × Female + 0.173 × Married - 0.218 × (Female × Married),
(0.075)(0.080)(0.097)
R2 = 0.44,SER = 0.375
Repeat the exercise in (e)of calculating the various percentage differences between gender and marital status.

Discern the importance of social and physical environments in child development through ecological theory.

Distinguish different research methodologies used in child development studies.

Understand the implications of research findings for correlational studies.

Differentiate between various developmental and psychological theories.

---

Definitions:

STI

Sexually Transmitted Infection, a contagious disease transmitted primarily through sexual contact.

Minor

An individual under the age of legal adulthood; also refers to something of lesser importance or size.

HIPAA Privacy Rule

A standard established to protect individuals' medical records and other personal health information, ensuring confidentiality within the healthcare sector.

Disclosure

The release of, the transfer of, the provision of access to, or the divulgence in any manner of patient information.