Let 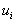Be Distributed N(0, ),I

question 53

Essay

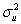Let be distributed N(0, ),i.e. ,the errors are distributed normally with a constant variance (homoskedasticity).This results in 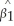being distributed N(β1, 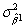),where 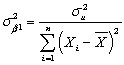.Statistical inference would be straightforward if 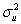was known.One way to deal with this problem is to replace 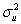with an estimator 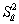.Clearly since this introduces more uncertainty,you cannot expect 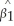to be still normally distributed.Indeed,the t-statistic now follows Student's t distribution.Look at the table for the Student t-distribution and focus on the 5% two-sided significance level.List the critical values for 10 degrees of freedom,30 degrees of freedom,60 degrees of freedom,and finally ∞ degrees of freedom.Describe how the notion of uncertainty about 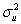can be incorporated about the tails of the t-distribution as the degrees of freedom increase.

---

Definitions:

Follicular Phase

The stage of a woman's menstrual cycle during which follicles in the ovary mature, ending with ovulation.

Placenta

An organ that develops in the uterus during pregnancy, providing oxygen and nutrients to the growing baby while removing waste products from the baby's blood.

IUD

An intrauterine device, a small contraceptive device inserted into the uterus to prevent pregnancy.

Birth Control

Methods, devices, or medications used to prevent pregnancy, allowing individuals or couples to determine the timing and spacing of childbirth.