At the Stock and Watson (Http://www

question 47

Essay

At the Stock and Watson (http://www.pearsonhighered.com/stock_watson)website go to Student Resources and select the option "Datasets for Replicating Empirical Results." Then select the "California Test Score Data Used in Chapters 4-9" (caschool.xls)and open it in a spreadsheet program such as Excel.
In this exercise you will estimate various statistics of the Linear Regression Model with One Regressor through construction of various sums and ratio within a spreadsheet program.
Throughout this exercise,let Y correspond to Test Scores (testscore)and X to the Student Teacher Ratio (str).To generate answers to all exercises here,you will have to create seven columns and the sums of five of these.They are
(i)Yi, (ii)Xi, (iii)(Yi- 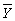), (iv)(Xi- 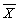), (v)(Yi- 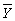)×(Xi- 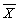), (vi)(Xi- 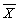)2, (vii)(Yi- 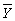)2
Although neither the sum of (iii)or (iv)will be required for further calculations,you may want to generate these as a check (both have to sum to zero).
a.Use equation (4.7)and the sums of columns (v)and (vi)to generate the slope of the regression.
b.Use equation (4.8)to generate the intercept.
c.Display the regression line (4.9)and interpret the coefficients.
d.Use equation (4.16)and the sum of column (vii)to calculate the regression R2.
e.Use equation (4.19)to calculate the SER.
f.Use the "Regression" function in Excel to verify the results.

Explain the formation and rights of a third-party beneficiary in a contract.

Recognize the limitations on the ability to delegate duties and assign rights.

Understand the implications of assignments that increase risk or burden.

Describe the rights of obligors and assignees after an assignment.

---

Definitions:

Ethnic Group

A community or population made up of people who share a common cultural background, including language, religion, and traditions.

Dominant Group

A social or ethnic group possessing a position of power, authority, or privilege, allowing them to set or influence societal norms and values.

Franklin Frazier

An influential African American sociologist known for his studies on the black family structure and race relations.

Matriarchal Patterns

Social or familial systems in which women, especially mothers, hold primary power and authority.