Math SAT Scores (Y)are Normally Distributed with a Mean of 500

question 4

Essay

Math SAT scores (Y)are normally distributed with a mean of 500 and a standard deviation of 100.An evening school advertises that it can improve students' scores by roughly a third of a standard deviation,or 30 points,if they attend a course which runs over several weeks.(A similar claim is made for attending a verbal SAT course. )The statistician for a consumer protection agency suspects that the courses are not effective.She views the situation as follows: H0 : 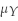= 500 vs.H1 : 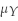= 530.
(a)Sketch the two distributions under the null hypothesis and the alternative hypothesis.
(b)The consumer protection agency wants to evaluate this claim by sending 50 students to attend classes.One of the students becomes sick during the course and drops out.What is the distribution of the average score of the remaining 49 students under the null,and under the alternative hypothesis?
(c)Assume that after graduating from the course,the 49 participants take the SAT test and score an average of 520.Is this convincing evidence that the school has fallen short of its claim? What is the p-value for such a score under the null hypothesis?
(d)What would be the critical value under the null hypothesis if the size of your test were 5%?
(e)Given this critical value,what is the power of the test? What options does the statistician have for increasing the power in this situation?

---

Definitions:

Output

The total amount of goods or services produced by a company, industry, or economy within a specified time period.

Total Cost Curve

A graphical representation showing the total cost of producing different levels of output in the short run or long run.

Quantity

The amount or number of units of a product, good, or service available or demanded in a market.

Fixed Input(s)

Factors of production, such as land or machinery, that cannot be adjusted in the short term.