Instruction 12-11
a Computer Software Developer Would Like to Use

question 163

Short Answer

Instruction 12-11
A computer software developer would like to use the number of downloads (in thousands)for the trial version of his new shareware to predict the amount of revenue (in thousands of dollars)he can make on the full version of the new shareware.Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different sharewares that he has developed:
$\begin{array}{lr}\hline {\text { Regression Statistics }} \\\hline \text { Multiple R } & 0.8691 \\\hline \text { R Square } & 0.7554 \\\hline \text { Adjusted R Square } & 0.7467 \\\text { Standard Error } & 44.4765 \\\text { Observations } & 30.0000 \\\hline\end{array}$
ANOVA
$\begin{array}{lr|r|r|r|r}\hline & d f & {\text { SS }} &{\text { MS }} & F & \text { Significance } F \\\hline \text { Regression } & 1 & 171062.9193 & 171062.9193 & 86.4759 & 0.0000 \\\hline \text { Residual } & 28 & 55388.4309 & 1978.1582 & & \\\hline \text { Total } & 29 & 226451.3503 & & & \\\hline\end{array}$

$\begin{array}{lrrrrrrr}\hline & \text { Coefficients } & \text { Standard Emor } & t \text { Stat } & \text { P-value } & \text { Lower 95\% } & \text { Upper 95\% } \\\hline \text { Intercept } & -95.0614 & 26.9183 & -3.5315 & 0.0015 & -150.2009 & -39.9218 \\\text { Download } & 3.7297 & 0.4011 & 9.2992 & 0.0000 & 2.9082 & 4.5513 \\\hline\end{array}$
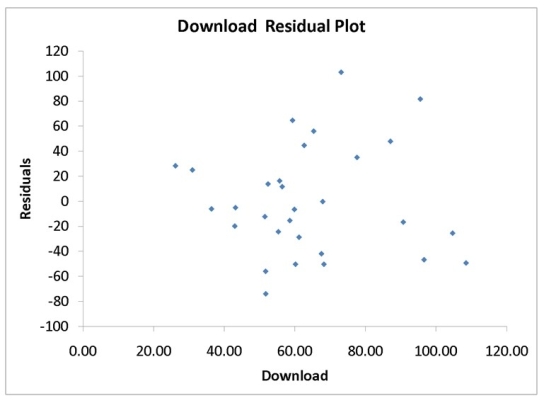
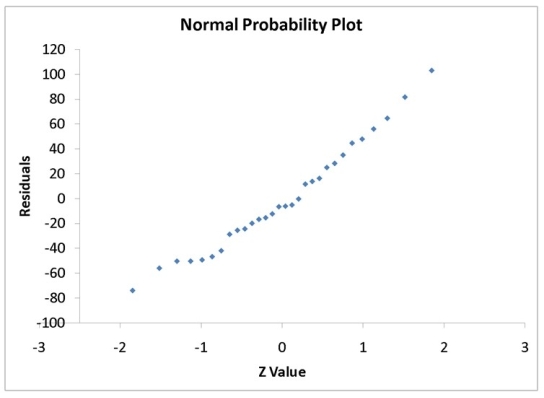
-Referring to Instruction 12-11,what is the standard error of estimate?

---

Definitions:

Variable Expense

Expenses that vary directly with changes in production volume or business activity levels.

Contribution Margin Ratio

A ratio that measures the proportion of sales revenue that exceeds variable costs, indicating how effectively a company can cover its fixed costs.

Target Profit

The desired net profit a company aims to achieve for a specific period.

Monthly Fixed Expense

Costs that do not vary in total regardless of production volume or sales levels, paid on a monthly basis.