Figure 1 Illustrates an Election in Which There Are Seven

question 8

Multiple Choice

Figure 1 illustrates an election in which there are seven voters (A, B, C, D, E, F, G) arrayed along a single left-right issue dimension that runs from 0 (most left) to 10 (most right) . Each voter is assumed to have a single-peaked preference ordering over the issue dimension and to vote for the party that is located closest to her ideal point. The voters are participating in a majority rule election in which there are two parties, P1 and P2, competing for office. These parties can be thought of as "office-seeking" parties since they only care about winning the election and getting into office.
Figure 1: Illustrating the Median Voter Theorem

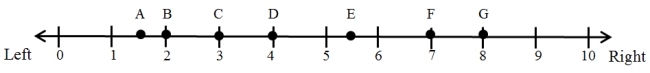
-Let's suppose that P₁ locates at Position 2 on the left-right issue dimension and that P₂ locates at Position 7. Who wins the election in the situation illustrated by Figure 1?

• A) The two parties tie.
• B) P₁ wins.
• C) P₂ wins.

---

Definitions:

Iterator

An object that enables the traversal of a container, particularly lists and sets, and allows the programmer to access each element in the container without exposing its underlying structure.

Container

In programming, a structure that holds multiple elements, often of similar types, providing various methods for storage and retrieval.

For-each Loops

A control flow statement for traversing items in a collection or array, providing a simpler and more readable way to iterate through the elements without using an index variable.

Collection Classes

Classes in programming that are used to store and manage groups of objects, such as lists, sets, and maps.